If you’re coloring in a map of, say, the United States and want to use as few colors as possible, but you don’t want two neighboring states to look the same… how few colors can you use? 10? 8? 5?
This is one of my favorite mathematical curiosities, and it’s simple enough for an elementary-aged student to work with, but also a legitimate puzzlement that has baffled professional mathematicians for decades.
First, I clarify what “neighboring” means. If two regions share a side, they can’t be the same color because it’s confusing. But, if they only share a point, that’s fine.

Of course, scaffolding is key, so you don’t start with a map with dozens of regions. You start with 3 or 4 regions. How few colors do you need for this map?
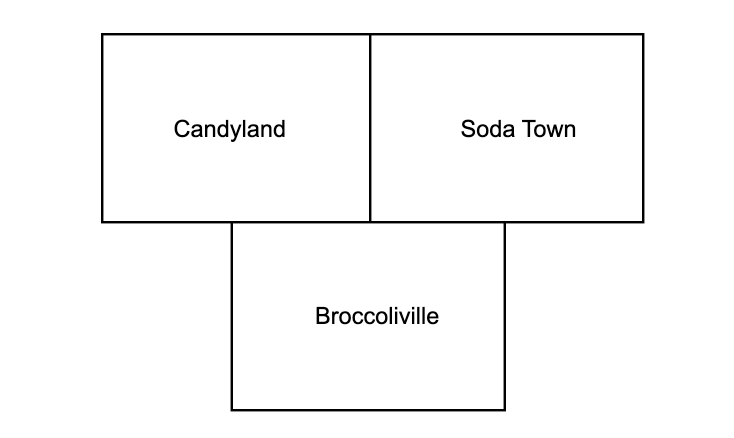
Then you add in some complexity. How few colors do you need for this map?
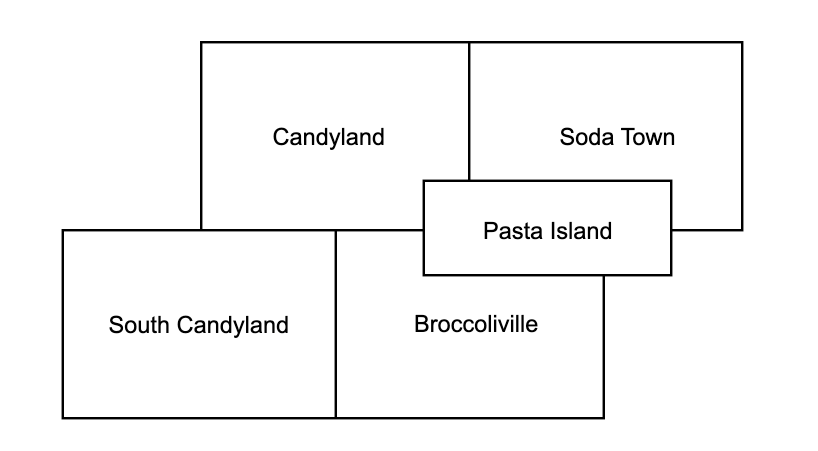
Move students towards a highly complex map. Perhaps students can create their own maps as well and test their hypothesis.
Eventually, you can reveal that no map needs more than four colors! In fact, this is actually called the four-color theorem (but don’t share that too early since it gives the answer away!)
Complex tasks like this are the key to a differentiated classroom. First, it’s complex enough to keep even my most advanced students engaged. Plus, while everyone is working away at this, I’m pulling groups to meet with (but, of course, not just my struggling students). And I don’t need to create three versions of this task, either. Everyone can work on it because of the scaffolding. As my colleague Lisa Van Gemert says, start with your most highly-able learner and scaffold down. For too long, I’d start “on-level” and try to add complexity. That just doesn’t work!
